Scientists are taught early on that when conducting measurements, one
must perform repeated experiments and average the results. If one
makes ![]() independent measurements of some observable
independent measurements of some observable ![]() ,
one computes the mean value as
,
one computes the mean value as
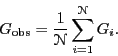 |
(6) |
We now imagine that the time of a measurement is so short that we know that the system is in only one of its many possible microstates.
This means we can write
Now we have to imagine that our system is evolving in time. As it
evolves, its degrees of freedom change values, and the system is
thought of as tracing out a trajectory in state space. (``State
space'' is a Hilbert space spanned by all states
![]() in
the quantum mechanical case, or phase space in the classical case.)
How is the system evolving? The system wavefunction evolves according
to Schrödinger's equation, while particles in a classical system
follow Newtonian mechanics. (We will consider Newtonian mechanics in
much greater detail later in Sec. 4.) As the
experimenters, we control the system by specifying a handful of
variables, such as its total energy,
in
the quantum mechanical case, or phase space in the classical case.)
How is the system evolving? The system wavefunction evolves according
to Schrödinger's equation, while particles in a classical system
follow Newtonian mechanics. (We will consider Newtonian mechanics in
much greater detail later in Sec. 4.) As the
experimenters, we control the system by specifying a handful of
variables, such as its total energy, ![]() , the number of particles,
, the number of particles,
![]() , and the volume,
, and the volume, ![]() . These contraints force the system's
trajectory to remain on a hyperdimensional surface in state space.
. These contraints force the system's
trajectory to remain on a hyperdimensional surface in state space.
The key assumption we make at this point is that, if we wait long
enough, our system will visit every possible state; that is, the
trajectory will eventually pass through every available point in state
space consistent with our constraints. If this is true, and we make
![]() independent observations, then the number of times we
observe the system in state
independent observations, then the number of times we
observe the system in state ![]() divided by the number of
observations,
divided by the number of
observations, ![]() , is the probability of observing
state
, is the probability of observing
state ![]() ,
, ![]() , if we happen to make a random observation. So,
Eq. 7 above becomes the ensemble average first
presented in Eq. 1:
, if we happen to make a random observation. So,
Eq. 7 above becomes the ensemble average first
presented in Eq. 1:
| (8) |
This assumption is important: it is referred to as the ergodic hypothesis. A system is ``ergodic'' if, after a sufficiently long time, it visits all possible state space points consistent with whatever constraints are put on it. We cannot in general prove that any system is ergodic; it is something we are comfortable assuming for most systems based on our physical intuition. There are, however, many systems which are non-ergodic. For the most part, we will not concern ourselves with such systems in this course.
Another important consideration is the following: How far apart must
the ![]() independent measurements be from one another in time
to be considered truly ``independent''? To answer this question, we
must introduce the notion of a relaxation time,
independent measurements be from one another in time
to be considered truly ``independent''? To answer this question, we
must introduce the notion of a relaxation time,
![]() , which arises naturally due to the presumably chaotic nature
of the microscopic system. Given some initial conditions, after a
time
, which arises naturally due to the presumably chaotic nature
of the microscopic system. Given some initial conditions, after a
time
![]() has elapsed, the system has ``lost memory'' of
the initial condition. We measure this loss of memory in terms of
correlation functions, which will be discussed in more detail
later. If we wait at least
has elapsed, the system has ``lost memory'' of
the initial condition. We measure this loss of memory in terms of
correlation functions, which will be discussed in more detail
later. If we wait at least
![]() between successive
observations, we know they are independent. It turns out that one can
use simulation methods to estimate relaxation times (and their
spectra; many systems display a broad spectrum of relaxation times,
each element cooresponding to a particular type of molecular motion).
We will pay particularly close attention to
between successive
observations, we know they are independent. It turns out that one can
use simulation methods to estimate relaxation times (and their
spectra; many systems display a broad spectrum of relaxation times,
each element cooresponding to a particular type of molecular motion).
We will pay particularly close attention to
![]() in
upcoming sections.
in
upcoming sections.